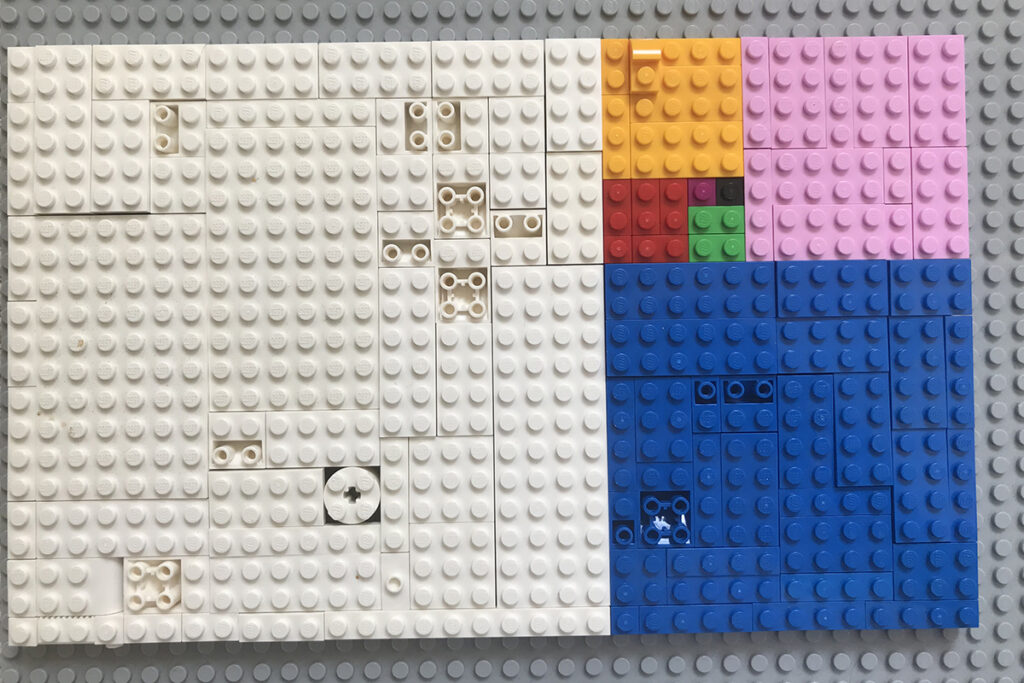The Fibonacci Sequence is a beautiful mathematical pattern that is easily accessible to children. The only skill needed to understand the sequence is basic addition, but diving into the life of Fibonacci and the sequence itself can help children see the connections between history, math, and nature. And it will help them begin to search for patterns all around them.
We began learning about the Fibonacci Sequence through the book, Blockhead: The Life of Fibonacci.

This children’s biography of Leonardo Fibonacci is incredibly rich in content. It tells the story of a boy following his dreams and love of numbers — despite being discouraged and ridiculed by his community — to discover a mysterious pattern in nature. It is an inspiring story, full of humanity, about overcoming adversity and being true to oneself.
In terms of math content, the book not only illustrates the Fibonacci sequence, but also touches on the concept of different number systems, as a young Fibonacci pushes to abandon Roman Numerals in favor of the Hindu-Arabic number system. In this way, the book not only describes mathematical concepts, but more importantly, presents mathematics as an artform that people across cultures and time have worked to develop.
Other Books

Growing Patterns, by Sara C Campbell
Stunning photographs from nature illustrate the elegance of the Fibonacci sequence.

Swirl by Swirl, by Joyce Sidman and Beth Krommes
Poetic verse and intricate illustrations show where spirals are found and how they function in nature.

The Rabbit Problem, by Emily Gravett
A story about Fibonacci’s rabbit problem, in which a rabbit population grows at an alarming rate! The illustrations are hilarious and entertaining as the story walks kids through the modeling of a famous math problem.
Activities
Modeling The Rabbit Problem
Within its story, Blockhead describes Fibonacci’s famous rabbit problem. In this problem, you start with a pair of baby rabbits. After one month, the pair of babies grow up, and become a pair of adults. After two months, the adult rabbits have a pair of babies. Each month after that, each existing pair of babies grows into an adult; and each existing pair of adults has a pair of babies. The question is, after a year, how many rabbits are there?

We represented the rabbits in this problem using pom poms, and tracked the first 6 months. Then we used the pattern to calculate the rest. This activity is more about learning how to model a problem than about finding the answer. Older kids might prefer to draw a tree or diagram instead of using manipulatives.

Month 1 – Pair of Babies 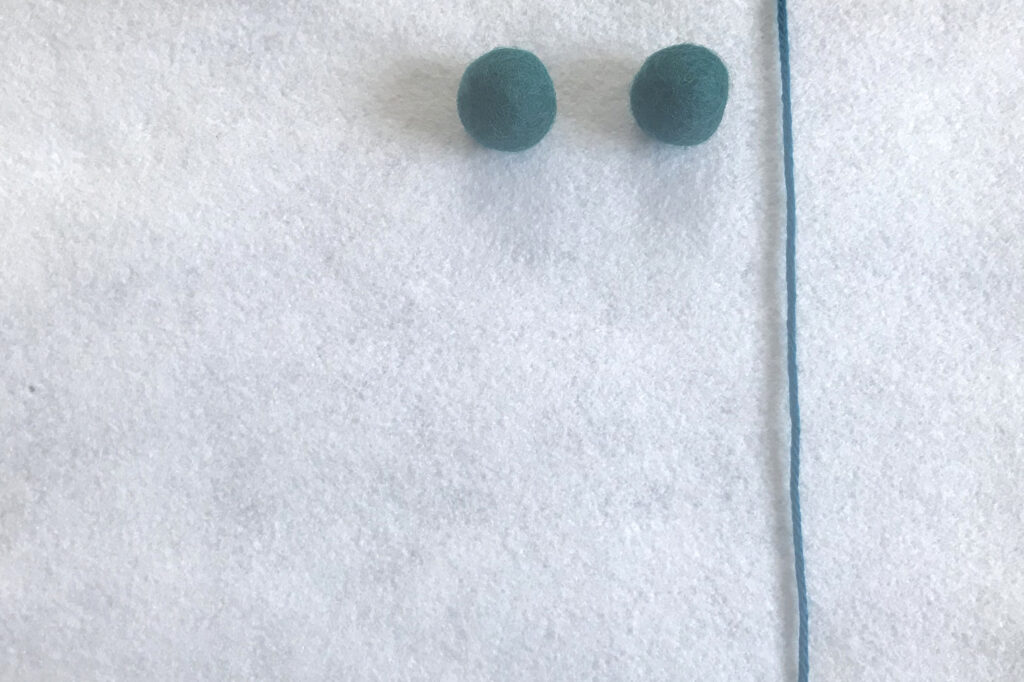
Month 2 – Pair of Adults 
Month 3 – Adults Age + New Babies 
Month 4 
Month 5 
Month 6 
Month 7
Nature Walks — Discovering Fibonacci
Since the Fibonacci sequence is found throughout nature, we searched for the pattern on nature walks. We counted the nodes on pinecones and the petals on flowers. If your child enjoys nature journaling, this is a great activity to cap off this nature walk. For us, just walking and making observations was enough.
A LEGO Drawing — Creating the Spiral
Turns out, we found even another use for LEGOs. We decided to build the Fibonacci spiral using LEGOs (apparently we inverted the spiral…)
This was harder than I thought it would be, because the number of LEGOs we needed for each color grew very quickly. It provided a great opportunity, though, to talk about how some sequences grow very quickly while others grow slowly.
If your child enjoys drawing and coloring, an alternative to this would be to color the pattern on graph paper.
Extensions
Patterns
If your child enjoys the Fibonacci sequence, this is a great time to look at other general math patterns and sequences. I used this opportunity to get a little basic math skills practice in, using pattern worksheets. You can find tons of free worksheets at DadsWorksheets.
Roman Numerals and Number Systems
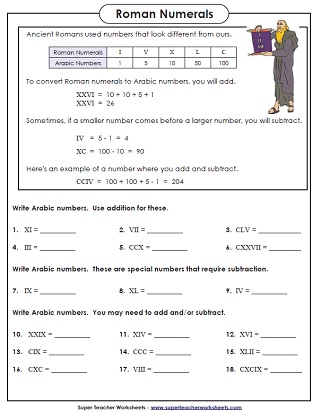
Since my son has studied Ancient Rome in one of his history projects, he was interested in learning about Roman Numerals in more depth. We practiced learning Roman Numerals with these sheets, from Super Teacher Worksheets, and then discussed the advantages and disadvantages of the Roman system as compared to the Hindu-Arabic number system.